Cuban Journal of Forest Sciences. 2021; January-Arpril 9(1):124-139

Translated from the original in spanish

Preliminary form and quotient factor of Brachystegia floribunda Benth.

Factor y cociente de forma preliminar de Brachystegia floribunda Benth.

Fator de forma preliminar e quociente de forma de Brachystegia floribunda Benth.

Edilio Aldana Pereira^1* https://orcid.org/0000-0001-8891-9799

Yordan Lores Pérez² https://orcid.org/0000-0002-8252-4590

¹University of Pinar del Río "Hermanos Saíz Montes de Oca". Pinar del Río, Cuba.
²Universidad de Guantánamo, Cuba.

^*Corresponding author: aldana@pap.upr.edu.cu

Received:17/7/2020.
Approved:11/12/2020.

ABSTRACT

One of the most important and widely used species in the natural forest of Miombo, in the Central Highlands of Angola, Brachystegia floribunda Benth is found mixed with other important species of the genera Brachystegia, Isoberlínea and Julbernardia, among others, but occupying the largest percentage of the forest area; however, there is limited accurate knowledge regarding the estimation of its commercial volume. This research, carried out in the forest areas of the Miombo formation, had the objective of determining the shape factors of the trunk and branches, as well as the shape quotient or diametric factor of the trunk. A sample of 53 trees, felled for logging and rigorously cubed, was used. The volumetric shape factor was determined, with and without grouping the trees in diameter classes. For the branches, only the volumetric shape factor was determined, with and without grouping, in branch length classes. As a final result, it was concluded that the volumetric and diameter shape factor of the trunks had a better correlation with the diameter, grouped in classes; and the correlation of the volumetric shape factor of the branches was higher when grouped in length classes.

Keywords: Volumetric estimate; Form factor; Form quotient; Brachystegia floribunda.

RESUMEN

Brachystegia floribunda Benth es una de las especies de mayor importancia y uso del bosque natural de Miombo, del Planalto Central de Angola, encontrándose mezclada con otras especies también importantes de los géneros Brachystegia, Isoberlínea y Julbernardia, entre otros, pero ocupando el mayor porcentaje de la superficie forestal; no obstante, el conocimiento veraz referente a las estimaciones de su volumen comercial es limitado. Esta investigación, ejecutada en las áreas forestales de la formación de Miombo, tuvo como objetivo, determinar los factores de forma del fuste y ramas, así como el cociente de forma o factor diamétrico del fuste. Se empleó una muestra de 53 árboles, derribados para aprovechamiento forestal y cubicado rigurosamente. Se determinó el factor volumétrico de forma, con y sin agrupamiento de los árboles en clases de diámetros. Para las ramas, solo se determinó el factor volumétrico de forma, con y sin agrupamiento, en clases de longitud de las mismas. Como resultado final se concluye que el factor volumétrico y diamétrico de forma del fuste tienen mejor correlación con el diámetro, agrupados en clases; y la correlación del factor volumétrico de las ramas es mayor cuando se agrupan en clases de longitud.

Palabras clave: Estimaciones; Volumétricas; Factor de forma; Cociente de forma; Brachystegia floribunda.

RESUMO

Brachystegia floribunda Benth é uma das espécies mais importantes e utilizadas na floresta natural de Miombo, no planalto central de Angola, misturada com outras espécies importantes dos géneros Brachystegia, Isoberlínea e Julbernardia, entre outros, mas ocupando a maior percentagem da área florestal; no entanto, o verdadeiro conhecimento sobre as estimativas do seu volume comercial é limitado. Esta investigação, realizada nas zonas florestais da formação Miombo, teve como objetivo determinar os fatores de forma do tronco e dos ramos, bem como o quociente de forma ou o fator diamétrico do tronco. Foi utilizada uma amostra de 53 árvores, abatidas para abate e rigorosamente cortadas em cubos. O fator de forma volumétrica foi determinado, com e sem agrupamento das árvores em classes de diâmetro. Para os ramos, apenas foi determinado o fator de forma volumétrica, com e sem agrupamento, em classes de comprimento. Como resultado final, conclui-se que o fator volumétrico e o diâmetro do tronco têm uma melhor correlação com o diâmetro, agrupados em classes; e a correlação do factor volumétrico dos ramos é maior quando são agrupados em classes de comprimento.

Palabras chave: Estimativas; Volumétrica; Factor de forma; Quociente de forma; Brachystegia floribunda.

INTRODUCTION

Brachystegia floribunda Benth is commonly known by ossasa and also by sassa in other regions of Angola (Sanfilippo, 2014). It is a tree whose trunk is straight and cylindrical, 6 -19 cm in diameter at the base, used, mainly, in the production of good quality charcoal, firewood, housing construction and wooden art objects.

The Miombo open forest occupies about 45.2 % of the total forest area of Angola, scattered over vast areas of the country and presents innumerable dominant associations, the most frequent being the genera Isoberlínea, Brachystegia and Julbernardia; Brachystyergia speciformis, Brachystegia tamarindoides, Brachystyergia floribunda, Brachystegia boehmii, Brachystegia utilis, Julbernardia paniculata and Ficus sansibarica.

The Miombo formation is of medium productivity in terms of commercial timber, but has a high social value in terms of woody fuel, construction materials, food products and medicinal plants (Caetano 2012). Several authors have conducted research work in this forest formation (Diniz 1973, Van Wyk 2013, Sardinha 2008, Figueiredo and Smith 2014, Sanfilippo 2013).

Brachystegia floribunda Benth is the subject of this research work. According to Péllico (2004) there is ample information on tree volumetry based on mathematical methods. Volumetric equations are one of the most efficient procedures for estimating the volume of standing trees. The equations do not always fit all forest species and forest population conditions, and it is advisable to test them and choose the model with the best result (Thomas 2006).

If the volume of a tree is correctly determined, the value found is valid for another tree of the same diameter, height and shape (Thiersch et al. 2006).

The real volume of a tree is the percentage of the volume of a cylinder, whose definition is given by the diameter at a height of 1.3 m from the ground and by the total or commercial height of the trees. Thus, this relationship between the volumes is called Shape Factor. According to the same author, the volume of a tree can be estimated by multiplying the cylindrical volume by an average Shape Factor appropriate to the species.

There is little information on research on volumetric and diametric factors of trunk shape for broadleaf species, whether natural or planted.

Therefore, the scientific problem of this research is related to the almost total absence of dendrometric and dasometric information on the main forest species of Miombo. Hence the need to begin research on the subject related to the shape of the trunk, in the species under study.

The objective is to determine the volumetric and diametric factors of trunk and branch shape, as well as their validation in the species Brachystegia floribunda.

MATERIALS AND METHODS

A total of 66 trees were felled and rigorously cubed, of which 53 were used to calculate the factors and shape quotients, and 13 were used to validate the results obtained.

The diameter variable was measured at the base of the trunk, at 0.3 m, at 0.5 m, at 1.3 m, at 1.5 m and every 0.5 m thereafter. Other variables measured were: total tree height, commercial height to the base of the canopy (trunk) and diameter at half the total length (d_0.5H). The same measurements were also made on the branches of the trees, but at the base of the branch (d_b), at 0.3 m (d_0.3) and at 1 m (d_1.0) and from here at 0.5 m to the end of the branch, and in addition, the diameter was measured at half the length of the branch (d_0.5H), and the diameter at half the length of the branch (d₀._5H). (Figure 1).

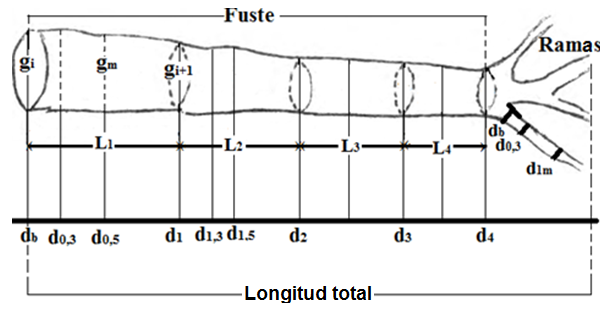
Figure 1. - Diagram of the diameter measurement in the felled tree

The method used was that of absolute sections, and the volume was calculated by means of the formula described by Newton (Equation 1).

Where:
V_i = Section volume i;
g_i = Larger end section area;
g_m = Area at mid-section;
g_i+1 = Minor end section area;
L_i = Section length.

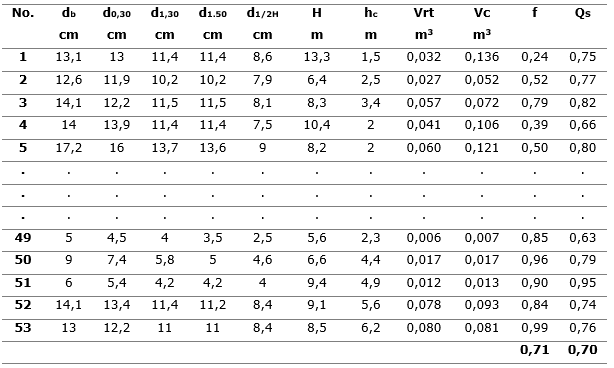
The databases where the dendrometric variables with bark are recorded, obtained for the trunk and branches respectively of the 53 trees sampled, are in the personal file of the authors. Table 1 only shows an outline of the database with some values.

Table 1. - Data base of the trunk with bark of the 53 sample trees of Brachystegia florinunda

To calculate the shape factor, the following equation was used (Equation 2) and (Equation 3).

The Schiffel method was used to calculate the shape quotient or diametric shape factor, i.e.: (Equation 4).

Where:
d_(1/2h) = diameter at half tree height;
d_1.3 = diameter at 1.3 m from the ground;
Q_s = Schiffel shape quotient.

Thus, the volume estimate will be (Equation 5).

Where:
DAP = d_1.3;
h = tree height;
Qs = Schiffel shape quotient.

To determine the validation statistics, the main statistics used were the coefficient of determination (R²), the standard error of the estimation, the standard error of the estimation, and the standard error of the estimation. (EEE). These statistics were estimated as follows (Equation 6):

Where:
Y₁ = observed value or the dependent variable;
Y₁ = average value of observed data;
Y₁ = predictive value;
p = number of model parameters including the intercept.

RESULTS AND DISCUSSION

In the last two columns of Table 1 are the mean values of the shape factor with bark for individual trees of B. floribunda and in the last row are the mean values of the volumetric shape factor f_1.3=0.71 and the diametric shape factor Q_S=0.70. There is practically no deference between the two factors. As shown in Figure 2, the volumetric shape factor has a slight decreasing trend with respect to increasing diameters.

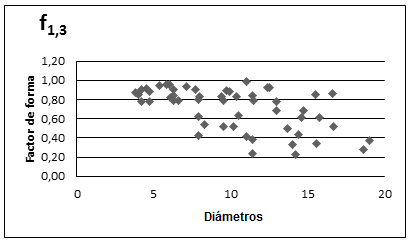
Figure 2. - Correlation of shape factor with respect to tree diameter

The trend of the correlation f_1,3/d_1,3 was analyzed with 5 types of correlation equations (logarithmic, linear, potential, exponential and polynomial) with the individual data of the sampled trees without any grouping. In all the equations there is a decreasing trend of f_1,3 with respect to diameters, but the coefficient of determination (R²) is very low, although the highest correlation is shown by the linear equation with an R² = 0.3176 and the second degree polynomial with an R²=0.3181 (Figure 3).

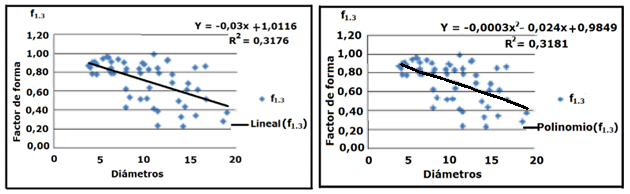
Figure 3. - Equations of higher correlation f_1,3/d_1,3 without grouping into diameter classes

But the graphical representation of the correlation f_1,3/d_1,3 fits better to the equation of a second degree polynomial.

Table 2 summarizes the mean values of f_1,3 grouped into diameter classes and also shows a clear decreasing trend with increasing diameters. But from diameter class 12 onwards the mean values of f_1,3 are lower than the mean value of f_1,3 =0.71 without grouping.

Table 2. - Volumetric factor (f_1,3) mean and by diameter classes for B. floribunda

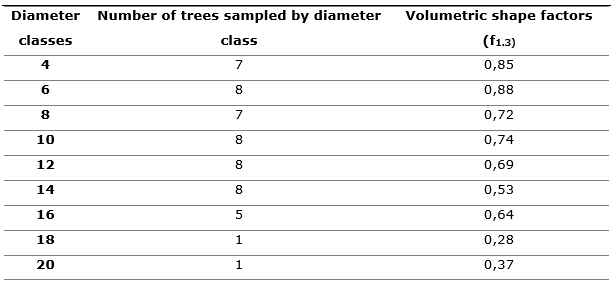
Figure 4 also presents the correlation equations f_1,3/d_1,3 and the trends of f_1,3 with respect to diameters and R² value grouped in diameter classes, with the same types of equations considered above, without grouping the values of f_1,3 by diameter classes.

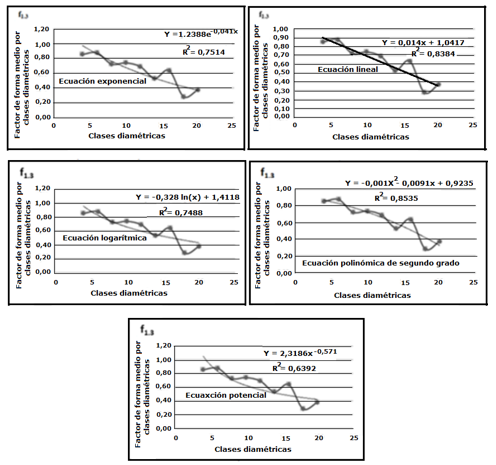
Figure 4. - Correlation equations f_1,3/d_1,3 grouping in diametric classes

Here it shows a more accentuated decreasing trend of and the coefficient of determination is higher than when f_1,3 values are not grouped in diameter classes, indicating that the correlation f_1,3/d_1,3 is higher when grouped in diameter classes. Here the highest correlation is also in the linear equations with an R² = 0.8384 and the second degree polynomial equation with an R² = 0.8535, higher than the linear equation.

Therefore, the graphical representation of the correlation f_1,3/d_1,3 grouped in classes fits better to the equation of a second degree polynomial.

Validation of the volumetric trunk shape factor of B. floribunda.

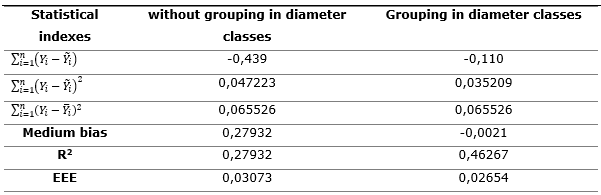
Table 3 shows the validation statistics for both shape factors (grouped and ungrouped). Although both R² are low, it was the volume estimated with the shape factor with clustered trees that showed the highest coefficient of determination. (R² = 0,46267). Also the lowest estimated standard error (EEE = 0.02654) was achieved with f_1,3 = 0.63 grouped in diameter classes and registered a low negative bias, therefore, it is advisable to use as a shape factor for the estimation of the standing tree volumes of B. floribunda, those grouped in diameter classes.

Table 3. - Validation statistics of the f_1,3 of B. floribunda.

Therefore, the volumetric shape factor to be used, preliminarily, for the estimation of the volume of the trunk of the species B. floribunda will be taken from Table 2 for each of the diameter classes.

Shape ratio of the trunk of B. floribunda

As shown in Figure 5, there is no marked trend of variation of the shape quotient with respect to diameters, also indicating a low correlation. Q_S/d.

The average shape quotient (QS) without grouping the trees by diameter classes, as shown at the end of the last column of Table 1, is 0.70; similar to the value of the volumetric shape factor, which is 0.71.

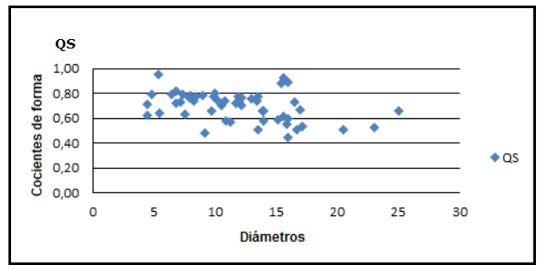
Figure 5. - Dispersion of the diameter shape factor or shape ratio (QS) with respect to the ungrouped diameters.

Table 4 shows the QS values when trees are grouped into diameter classes, where a decreasing trend is observed with increasing diameter. This trend corresponds to that shown by the volumetric trunk shape factor, which means that, although there is a difference in values of 0.04, in practice they are equivalent to each other.

Table 4. - Trunk shape ratios of Brachystegia floribunda Benth according to diametric classes

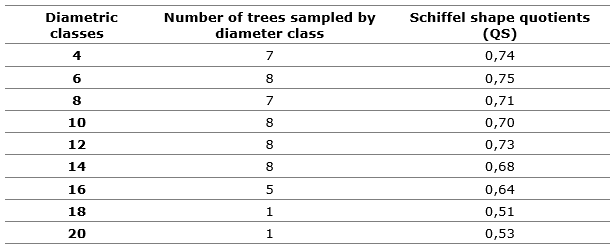
Figure 6 shows the equation defining the trend of the shape to diameter ratios.

The equation corresponds to a second degree polynomial with a high correlation of 0.945 and a given coefficient of R²=0,894, also high.

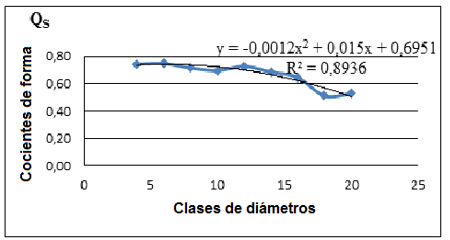
Figure 6. - Correlation (QS/d_{1, 3}) of the shape ratios of B. floribunda with respect to diameter classes

Volumetric shape factor of the branches of B. floribunda.

Table 5 also shows an outline of the database with some values, where the main dendrometric variables measured in each of the evaluated branches are indicated. The last three columns show the shape factors taking reference diameters at the base at 0.3 m from the base and at 1.0 m from the base.

Table 5. - Dendrometric variables and shape factors of B. floribunda branches

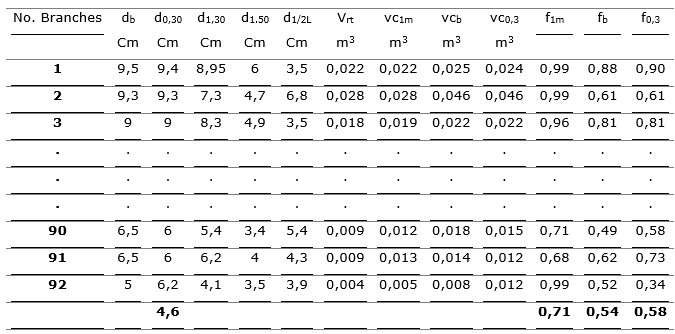
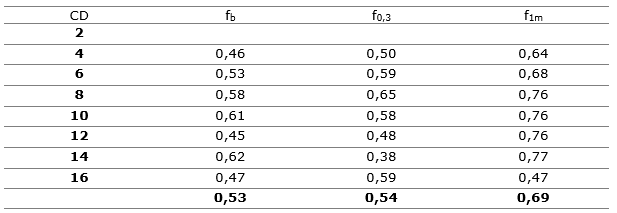
The shape factors f_b and f_0,3 show the greatest dispersion. The mean values of the shape factors of the ungrouped branches are as follows f_b = 0,54; f_0,3 = 0,58 e f_1m =0,71

Table 6 shows the results of the shape factors with the same reference diameters, but grouped by branch length class.

Table 6. - Shape factors of Brachystegia floribunda branches according to length class

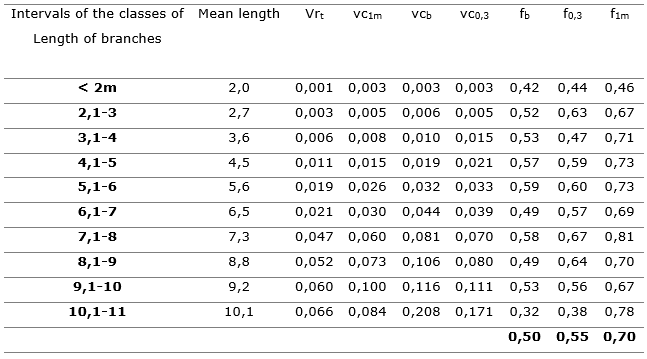
In this case, the shape factors grouped in length classes are: f_b = 0.50; f_0,3 = 0.55 and f_1m =0.70 respectively and were obtained from the branch that has the average length of the interval or range of all the trees in that class interval.

Figure 7 shows the correlation of the branch shape factors with respect to the ungrouped and grouped branch lengths.

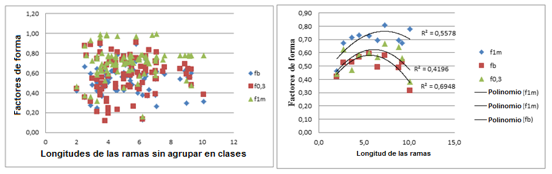
Figure 7. - Shape factors of branches with different reference diameters

The shape factor of the branches grows with the height of the reference diameter; the same happens when they are grouped in length classes. In addition, they increase with length up to about 6 or 7 meters, after which they begin to decrease.

The equation that best defined the trend line and the one that showed the highest correlation between the respective shape factors and branch length was the second degree polynomial; f_b had the highest correlation with branch length with a coefficient of determination of R²=0,6948.

The shape factors of the branches were also evaluated by grouping them by diameter clases (Table 7). In this case, the values obtained were: f_b = 0,53; f_0,3 = 0,54 y f_1m =0,69.

Table 7. - Branch shape factors in relation to diameter grouped into diameter classes

As shown in Figure 8, the shape factors grouped into diameter classes are similar to those obtained when grouped into length classes, except for the shape factor at the base (f_b) which is higher, while (f_0,3) and (f_1m) are slightly lower.

Figure 8 shows the trends and coefficients of determination of the three branch shape factors in relation to the diameter classes.

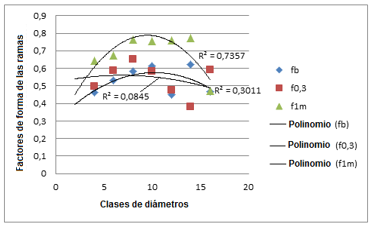
Figure 8. - Correlation of branch shape factors with length grouped into diameter classes

The equations of the three trend lines also correspond to a second degree polynomial and f_b was also the most highly correlated, with a coefficient of determination of 0.736, higher than the value when the shape factors are grouped into length classes.

In general it is observed that, while in the shaft the shape factor decreases with increasing diameter, in the branch it tends to increase with the diameter up to approximately 10 cm and from there it begins to decrease, i.e. the shape of the branches tends more to the shape of the paraboloid.

Validation of the volumetric shape factors of the branches of the species

Brachystegia floribunda

An analysis of the statistical indices allowed a more accurate evaluation of the best average shape factor for the estimation of the volume of the branches of Brachystegia floribunda.

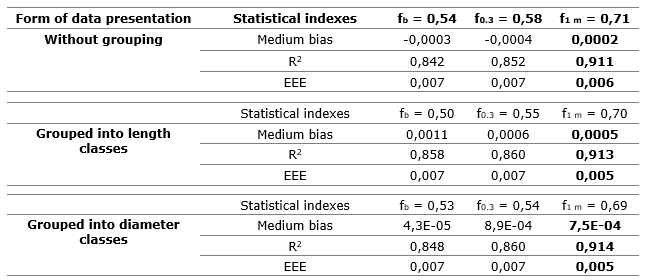
Table 8 shows that the mean shape factor calculated with the reference diameter at 1 m from the base of the branch had the highest correlation with the length of the branches and with the ungrouped diameters, but the best results were obtained when the branches were grouped in length or diameter classes, showing practically no difference between the two forms of branch grouping. Therefore, for the estimation of branch volume in Brachystegia floribunda it is recommended to measure the reference diameter to calculate the volume of the cylinder at 1 m from the base of the branches and multiply this volume by the shape factor of 0.7.

Table 8. - Validation statistics of the three form factors (f_b), (f_0,3) and (f₁) of the branch of Brachystegia floribunda

CONCLUSIONS

There is a correlation between shape factor and diameter, being higher when trees are grouped into diameter classes.

The shape factor decreases with increasing diameter, fitting a second degree polynomial equation, but the recommended value should be for each diameter class when trees are grouped into diameter classes.

The branch form factor of Brachystegia floribunda has a tendency to increase with the height of the reference diameter and branch length up to about 10 cm in diameter and up to about 6 or 7 meters in length, after which it begins to decrease.

To estimate the volume of Brachystegia floribunda branches, the reference diameter must be measured to calculate the volume of the cylinder at 1 m from the base of the branches and this volume must be multiplied by the shape factor of 0.69.

The average value of the shape quotient calculated without grouping the trees by diameter classes is 0.70, similar to the value of the volumetric shape factor.

It is recommended to use the shape quotient that corresponds to the diameter class of the tree for the volume estimation. To estimate the volume of Brachystegia floribunda branches, the reference diameter must be measured to calculate the volume of the cylinder at 1 m from the base of the branches and this volume must be multiplied by the shape factor of 0.69.

The average value of the shape quotient calculated without grouping the trees by diameter classes is 0.70, similar to the value of the volumetric shape factor.

It is recommended to use the shape quotient that corresponds to the diameter class of the tree for the volume estimation.

REFERENCES

ALBUQUERQUE SARDINHA, R.M. de, 2008. Estado, dinâmica e instrumentos de política para o desenvolvimento dos recursos lenhosos no município da Ecunha, Angola [en línea]. Angola: IMVF - Instituto Marquês de Valle Flôr. [Consulta: 3 septiembre 2020]. Disponible en: https://pdfslide.net/documents/desenvolvimento-dos-recursos-lenhosos-ecunha.html.

CAETANO, T.P., 2012. Perfil forestal de Angola. 1^o Congresso dos Engenheiros de Língua Portuguesa. Angola: s.n.

CASTANHEIRA DINIZ, A., 1973. Características mesológicas de Angola: descrição e correlação dos aspectos fisiográficos, dos solos e da vegetação das zonas agrícolas angolanas [en línea]. Angola: Missão de Inquéritos Agrícolas de Angola. ISBN 972-8975-02-3. Disponible en: https://books.google.com.cu/books/about/Caracter%C3%ADsticas_mesol%C3%B3gicas_de_Angola.html?id=f -c6AAAAMAAJ&redir_esc=y.

OLIVEIRA, A.D. de, DEHON, G., SCOLFORO, J.R. y THIERSCH, C.R., 2006. Acurácia dos métodos para estimación do volume comercial de clones d e Eucalyptus sp. Cerne, Lavras [en Línea], vol. 12, no. 2, pp. 167-181. [Consulta: 3 septiembre 2020]. Disponible en: https://www.researchgate.net/publication/237039557_Acuracia_dos_metodos_para_estimación_do_volume_comercial_de_clones_de_Eucalyptus_sp.

PÉLLICO NETTO, S., 2004. Equivalência volumétrica: uma nova metodologia para estimación do volume de árvores. Revista Acadêmica: ciências agrárias e ambientais, vol. 2, no. 1, pp. 17-30.

SANFILIPPO, M., 2014. Trinta árvores e arbustos do Miombo Angolano. Guia de campo para a identificação [en línea]. Angola: ONG COSPE (Cooperazione per lo Sviluppo ei Paesi Emergenti). [Consulta: 3 septiembre 2020]. Disponible en: https://www.academia.edu/17163898/Trinta_%C3%A1rvores_e_arbustos_do_miombo_Angolano_Guia_de_campo_para_a_identifica%C3%A7%C3%A3o.

THOMAS, C., ANDRADE, C.M., SCHNEIDER, P.R. y GUIMARÃES FINGE, C.A., 2006. Comparação de equações volumétricas ajustadas com dados de cubagem e análise de tronco. Ciencia Florestal Santa Maria [en línea], vol. 16, no. 3, pp. 319-327. [Consulta: 3 septiembre 2020]. ISSN 0103-9954. DOI 10.5902/198050981911. Disponible en: https://www.researchgate.net/publication/27790615_Comparacao_de_equacoes_volumetricas_ajustadas_com_dados_de_cubagem_e_analise_de_tronco.

WYK, B. Van, 2013. Field Guide to Trees of Southern Africa [en línea]. South Africa: Penguin Random House South Africa. ISBN 978-1-77584-104-3. Disponible en: https://books.google.com.cu/books/about/Field_Guide_to_Trees_of_Southern_Africa.html?id=dw1bDwAAQBAJ&redir_esc=y.

Conflict of interests:
The authors declare not to have any interest conflicts. 

Authors' contribution:
Edilio Aldana Pereira: Conception of the idea, literature search and review, instrument making, instrument application, compilation of information resulting from the instruments applied, statistic análisis, preparation of tables, graphs and images, database preparation, general advice on the topic addressed, drafting of the original (first version), review and final version of the article, article correction, authorship coordinator, translation of terms or information obtained, review of the application of the applied bibliographic standard.

Yordan Lores Pérez: Conception of the idea, literature search and review, instrument making, instrument application, compilation of information resulting from the instruments applied, statistic análisis, preparation of tables, graphs and images, database preparation, general advice on the topic addressed, drafting of the original (first version), review and final version of the article, article correction, authorship coordinator, translation of terms or information obtained, review of the application of the applied bibliographic standard.

This work is licensed under a Creative Commons Attribution-NonCommercial 4.0 International license
Copyright (c) 2021 Edilio Aldana Pereira, Yordan Lores Pérez.